Lionel Robbins famously argued that interpersonal comparisons of utility were meaningless due to the psychological and introspective nature of the concept of utility, a case of “thy blood or mine”. Samuelson introduced the idea of revealed preference to allow for a scientific theory of demand in response to such attacks on the notion of utility as a purely subjective non-observational concept. Samuelson’s project was thus to derive the major results of ordinal utility theory while relying on observable propositions. The theory of revealed preference, and the Weak Axiom of Revealed Preference (WARP), were designed to base demand theory on observed behavior. Mas Colell writes that Samuelson’s insight was to “enunciate the general methodological postulate that the basic axioms of a theory must be operational – that is, they must be refutable by observable data generated from feasible experiments.” (Mas Colell, 1982: 73). In this post we attempt to examine whether WARP and the theory of revealed preference have really achieved the purported result of placing the theory of consumer demand on a scientific footing.
1. Revealed Preference And The Weak Axiom of Revealed Preference
We find a definition of “revealed preference” in Def. 1.C.2 (MWG p.11)
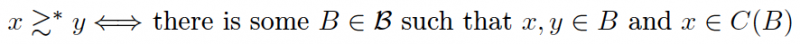
We say that x is “revealed as good as” y (we can write xRy) if x is observed to be chosen when both x and y are available from a budget set B. If it is the case that y is also not chosen frm B, we say that x is “revealed preferred to y” (we can write xPy). For this purpose, the set C(B) is defined as the set of chosen elements from a particular budget set B. The framework for choice-behavior is presented in MWG Ch.1 with a high degree of generality, allowing the choice set to contain more than one element (this generality is lost subsequently in Ch.2 — when the Walrasian demand function is presented as a choice rule for the consumer, it is taken to be single-valued).
There are three different versions of WARP formulated in MWG. All three are rigorously equivalent.
Definition 1.C.1 (MWG, p.10)
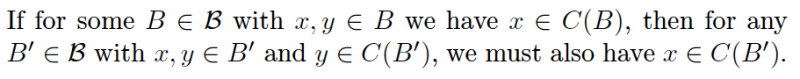
In short, if both x and y are available when x is chosen from a first budget set, it cannot be the case that y is subsequently chosen from a different budget set, with x also not being chosen if both x and y are available.
A restatement of this definition is found on MWG, p.11. We can restate WARP as follows. If x is revealed as good as y (xRy), then y cannot be revealed preferred to x (not yPx). This statement, of course, is also equivalent to Definition 1.C.1.[1]
Definition 1.C.1 can be used to derive another proposition (stated in Exercise 1.C.2):
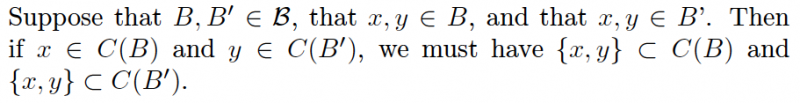
This proposition is essentially applying WARP to both x and y.
2. The Choice Rule
There are different possible interpretations for the fact that C(B) may contain more than one element. One possible interpretation is that we have the ability to look inside people’s mind to determine what they would consider acceptable. Yet this view might be problematic since the choice-based approach to decision-making is intended to reflect actual observed choice making. We might imagine that a complete snapshot of a consumer’s mindset at a single point in time could be assessed by presenting the consumer with various questionnaires soliciting an enumeration of all possible acceptable goods to be selected from a given budget set. Yet it is not very realistic to assume that choice sets be generated in this fashion. Further, a consumer’s assessment as to what they might choose in a given situation is not necessarily the same as actual choice.
Another possible interpretation (MWG’s interpretation) as to why the choice rule can specify more than one element is that the choice set contains all elements in B that the decision-maker might choose over time from a given budget set (MWG, p10). As such the set must be generated by repeated observations rather than any single observed occurrence of choice being exercised. This requires that we be able to repeatedly observe the agent repeatedly making choices from a given budget set over time. [We note, here, in passing the issue of assessing whether the commodities included in the budget set remain the same commodities if made available to the consumer at different points in time. In defining the commodity vector in the commodity space, MWG makes a point to note that theoretically “rigorously, bread today and tomorrow should be viewed as distinct commodities” (MWG, p.18)]
3. Falsifying the Revealed Preference Relations – Weak and Strict
Here we come to an epistemic issue. How many observations are necessary to conclude that we have established an exhaustive list of acceptable alternatives? How do we know when to stop observing? This point is critical if we are to test the falsifiability of some of the theory’s propositions. For instance, we can see that the claim xPy can be falsified — it is sufficient to observe that y is chosen when both x and y are available in B [i.e. we must observe that y is in C(B)]. For this purpose, one single observation is sufficient to falsify xPy — it is sufficient to establish that y was so chosen from B at least once in order to conclude that y is in C(B). It does not matter that 1,000 observations might record x being chosen over y prior to seeing y being chosen in the 1,001th observation.
Consider, on the other hand, the claim that xRy. What would be required to establish that this claim is false? There are only three possibilities:
- We might observe x (and only x) being chosen when both x and y are available. This observation is consistent with the claim xRy.
- We might observe both x and y being chosen – this observation is also consistent with the claim.
- Finally, we might observe y repeatedly being chosen over x (i.e. x is never chosen, but y is). Here we might be tempted to conclude that we have falsified xRy. However, can we rule out the possibility that x is indeed in the choice set, but we simply have yet to observe x being chosen? If we have observed the consumer choosing y over x every single time during 1,000 repeated trials, we might come to regard these repeated observations as some sort of inductive evidence for the proposition that x is not weakly preferred to y. Yet we have not falsified the claim that xRy. It is indeed possible that on the 1,001th trial, we will observe x being chosen from the budget set, and that the claim is true after all. Falsifying the weak preference relation is impossible unless we assume that there is some observational “cut-off point”, some maximum number of observations used to derive all elements in our choice set, beyond which we would simply discard any new data that might add elements to the set. The specific determination of what exact number of observations is sufficient to determine that the choice set is complete is, of course, a difficult one.
The indifference relation is also subject to the same falsification issue: in order to falsify the claim that x~y, we must produce evidence that we do not have both xRy and yRx. But we have just seen that this is impossible unless we somehow restrict ourselves to a limited number of observations.
4. Falsifying the Weak Axiom of Revealed Preference
Let us consider the falsifiability of WARP. WARP entails that if xRy, then we cannot have yPx. Falsifying WARP would require evidence that if x was chosen when y was available, it is possible to have yPx, i.e. that:
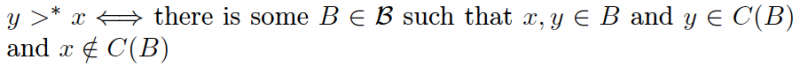
Here we run into the issue of showing that x is not in C(B), and when to satisfy ourselves that the choice set is complete (that we have a sufficient number of observations). Another way to think about this is to focus on the third formulation of WARP (stated in Exercise 1.C.2). If we observe x chosen from a budget set B, and y chosen from a different budget set B’, and x and y were available in both budget sets, a violation of WARP would entail that y is not in C(B), and / or x is not in C(B’). We come across the same issue of how to satisfy ourselves that our choice sets are complete. When can we be sure that y is never chosen from B, and x from B’? If we have observed the consumer 100 times, and y was never chosen from B, when can we conclude with certainty that y is indeed not an alternative that the consumer might choose?
5. Some Concluding Thoughts
In his original presentation of the concept of revealed preference, Samuelson’s approach was based upon two observations at two different time periods, which eliminates these issues: “if an individual selects batch one over batch two, he does not at the same time select two over one. The meaning of this is perfectly clear and will probably gain ready acquiescence.” (Samuelson, 1938: 65) There was no thorough discussion of what it means for an individual to “select” a batch, but the idea of the choice set containing more than one element, all the alternatives acceptable to the consumer, was not explicitly introduced.
The approach is quite basic and straightforward in this original version of WARP. Choice does appear to be defined through what the consumer actually chooses in a given instance rather than the somewhat more hypothetical view of what the consumer might choose (which entails the difficult issue of repeated choice experiments over time). Yet the approach is not without flaws, potentially. This definition of WARP does not properly accommodate for the possibility of indifference. As pointed out by Sen (1995), if there are more than one optimal alternatives between which the chooser is indifferent, x and y, he or she may choose x in the presence of y and y in the presence of x without necessarily contradicting himself or herself and acting irrationally. Yet WARP would be violated, and the behavior deemed internally inconsistent (See Sen, 1995:22). This is indeed somewhat bizarre.
This problem can be avoided if the choice rule is formulated so as to allow for the possibility that elements chosen from a set not be limited to what is actually chosen, but encompass all alternatives that might be chosen, which is exactly what the MWG definition of the choice rule achieves. Sen also contemplates this possible “rescue” of WARP: “Rather than taking the demand of maximization to be the choosing of some alternative that is not established as worse than any other, it could be demanded that all alternatives that are no worse than any other are recognized as ‘choosable’.” (Sen, 1995: 30 footnote 14). In this case, arguably if the consumer is truly indifferent between x and y, both x and y may be found in the choice set (if both are made available to the consumer), and WARP is not violated.
Hence the more encompassing MWG view eliminates issues of seemingly inconsistent behavior in the case of indifference. Yet it does so at the cost of introducing the issue of the unfalsfiability of WARP. One cannot have one’s cake and eat it too.
References
Mas-Colell, A. (1982): “Revealed Preference after Samuelson,” in G.R. Feiwel, ed., Samuelson and Neoclassical Economics, Amsterdam: Kluwer, pp.72–82.
Samuelson, P. (1938), “A Note on the Pure Theory of Consumer Behavior.” Economica 5(17): 61-71
Sen, A. (1995). Is the Idea of Purely Internal Consistency of Choice Bizarre? In James, E., Altham, J. and Harisson R., editors, World, Mind and Ethics: Essays on the Ethical Philosophy of Bernard Williams.Cambridge, UK: Cambridge University Press
[1] This follows from the definition of revealed preference. If “x is revealed as good as y”, we must have {x,y} element of B, and x element of C(B). This is the first half of Def. 1.C.1… Then we cannot have “y revealed preferred to x”: If x and y are subsequently available in another budget set, we cannot have y chosen but x not chosen. This corresponds to the second half of Def 1.C.1: if {x,y} element of B’, and y is element of C(B’), we must have x element of C(B’).




